The study of fluid dynamics is crucial in understanding various natural and industrial processes, including the flow of fluids in pipes, around objects, and through channels. One of the fundamental concepts in fluid dynamics is the distinction between laminar and turbulent flow. These two types of fluid flow exhibit distinct characteristics, influencing the behavior, efficiency, and application of fluid-based systems. In this article, we will delve into the principles, differences, and practical implications of laminar and turbulent flow, providing a comprehensive overview for professionals and enthusiasts alike.
Key Points
- Laminar flow is characterized by smooth, orderly layers of fluid, while turbulent flow involves chaotic, irregular motion.
- The Reynolds number, a dimensionless quantity, is used to predict the onset of turbulence and distinguish between laminar and turbulent flow regimes.
- Laminar flow is generally associated with lower Reynolds numbers and is more energy-efficient, but it can also lead to boundary layer separation and increased drag.
- Turbulent flow, on the other hand, is characterized by higher Reynolds numbers, increased mixing, and higher energy losses due to friction and vortex formation.
- Understanding the differences between laminar and turbulent flow is essential for designing and optimizing various systems, including pipelines, heat exchangers, and aerodynamic shapes.
Characteristics of Laminar Flow

Laminar flow, also known as streamline flow, is characterized by smooth, orderly layers of fluid that flow parallel to each other. This type of flow is typically observed at low velocities, low Reynolds numbers, and in narrow channels or pipes. The fluid particles in laminar flow follow well-defined paths, with minimal mixing or exchange of momentum between adjacent layers. As a result, laminar flow is often associated with lower energy losses, reduced friction, and increased efficiency.
Reynolds Number and Laminar Flow
The Reynolds number, a dimensionless quantity, is used to predict the onset of turbulence and distinguish between laminar and turbulent flow regimes. The Reynolds number (Re) is defined as the ratio of inertial forces to viscous forces and is calculated using the following equation: Re = ρUL/μ, where ρ is the fluid density, U is the average velocity, L is the characteristic length, and μ is the dynamic viscosity. For laminar flow, the Reynolds number is typically less than 2000.
| Reynolds Number Range | Flow Regime |
|---|---|
| Re < 2000 | Laminar flow |
| 2000 < Re < 4000 | Transition regime |
| Re > 4000 | Turbulent flow |
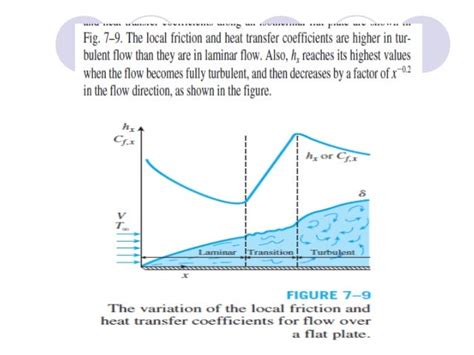
Characteristics of Turbulent Flow

Turbulent flow, on the other hand, is characterized by chaotic, irregular motion of fluid particles. This type of flow is typically observed at high velocities, high Reynolds numbers, and in wider channels or pipes. The fluid particles in turbulent flow exhibit random, three-dimensional motion, with significant mixing and exchange of momentum between adjacent layers. As a result, turbulent flow is often associated with higher energy losses, increased friction, and reduced efficiency.
Reynolds Number and Turbulent Flow
For turbulent flow, the Reynolds number is typically greater than 4000. The transition from laminar to turbulent flow occurs when the Reynolds number exceeds a critical value, which depends on the specific geometry and flow conditions. In turbulent flow, the fluid particles exhibit a wide range of velocities and directions, leading to increased mixing and energy losses.
Practical Implications and Applications
The differences between laminar and turbulent flow have significant implications for various engineering and industrial applications. For example, in pipeline design, laminar flow is often preferred due to its lower energy losses and increased efficiency. However, turbulent flow can be beneficial in certain applications, such as mixing and heat transfer, where increased mixing and energy exchange are desired.
Aerodynamic Applications
In aerodynamics, the distinction between laminar and turbulent flow is critical for designing efficient aircraft and wind turbine blades. Laminar flow is often desired over the surface of these objects to reduce drag and increase lift. However, turbulent flow can be beneficial in certain regions, such as near the trailing edge, where increased mixing and energy exchange can help to reduce drag and improve overall performance.
What is the main difference between laminar and turbulent flow?
+The main difference between laminar and turbulent flow is the nature of the fluid motion. Laminar flow is characterized by smooth, orderly layers of fluid, while turbulent flow involves chaotic, irregular motion.
What is the role of the Reynolds number in distinguishing between laminar and turbulent flow?
+The Reynolds number is a dimensionless quantity that is used to predict the onset of turbulence and distinguish between laminar and turbulent flow regimes. A low Reynolds number typically indicates laminar flow, while a high Reynolds number indicates turbulent flow.
What are the practical implications of laminar and turbulent flow in industrial applications?
+The distinction between laminar and turbulent flow has significant implications for various industrial applications, including pipeline design, heat transfer, and aerodynamics. Understanding the characteristics and implications of each flow regime is essential for optimizing system performance, reducing energy losses, and improving overall efficiency.
In conclusion, the study of laminar and turbulent flow is essential for understanding various natural and industrial processes. By recognizing the distinct characteristics and implications of each flow regime, engineers and researchers can design and optimize systems to achieve improved efficiency, reduced energy losses, and enhanced performance. Whether in pipeline design, heat transfer, or aerodynamics, the distinction between laminar and turbulent flow plays a critical role in shaping the behavior and efficiency of fluid-based systems.



